Fresnel zones are areas into which the surface of a sound or light wave is divided in order to calculate the results of diffraction of sound or light. For the first time this method was applied by O. Fresnel in 1815.
History reference
Augustin Jean Fresnel (10.06.1788-14.07.1827) - French physicist. He devoted his life to studying the properties of physical optics. Back in 1811, under the influence of E. Malyus, he began to study physics independently, and soon became interested in experimental research in the field of optics. In 1814, he “rediscovered” the principle of interference, and in 1816 he supplemented the well-known Huygens principle, in which he introduced the idea of coherence and interference of elementary waves. In 1818, based on the work done, he developed the theory of light diffraction. He introduced the practice of viewing diffraction from the edge, as well as from a circular hole. Conducted experiments that later became classical, with biprisms and bizzerkas on the interference of light. In 1821, he proved the fact of the transverse nature of light waves, in 1823 he discovered circular and elliptical polarizations of light. Explained on the basis of wave representations, chromatic polarization, as well as the rotation of the plane of polarization of light and birefringence. In 1823, he established the laws of refraction and reflection of light on a fixed flat surface between two media. Along with Jung is considered the creator of wave optics. He is the inventor of a number of interference devices, such as Fresnel mirrors or Fresnel biprism. He is considered the founder of a fundamentally new way of lighthouse lighting.
Bit of theory
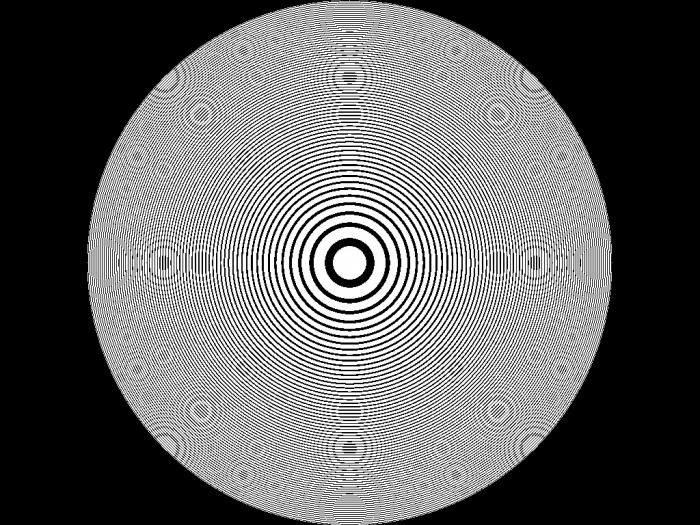
Fresnel zones can be determined both for diffraction with an aperture of arbitrary shape, and without it at all. However, from the point of view of practical expediency, it is best to consider it on a round hole. In this case, the light source and the observation point should be on a straight line that is perpendicular to the plane of the screen and passes through the center of the hole. In fact, any surface through which light waves pass can be divided into Fresnel zones. For example, surfaces of equal phase. However, in this case it will be more convenient to divide a flat hole into zones. To do this, we consider an elementary optical problem that will allow us to determine not only the radius of the first Fresnel zone, but also the subsequent ones with arbitrary numbers.
The task of determining the size of the rings
First, imagine that the surface of the flat hole is between the light source (point C) and the observer (point H). It is perpendicular to the CH line. The segment CH passes through the center of the circular hole (point O). Since our task has an axis of symmetry, the Fresnel zones will have the form of rings. And the decision will come down to determining the radius of these circles with an arbitrary number (m). In this case, the maximum value is called the radius of the zone. To solve the problem, it is necessary to make an additional construction, namely: select an arbitrary point (A) in the plane of the hole and connect it with straight line segments with the observation point and with the light source. As a result, we get the SAN triangle. Further, it can be done so that the light wave arriving at the observer along the SAN path will travel a greater path than the one that will follow the SN path. From this we obtain that the path difference CA + AN-CH determines the difference between the wave phases that passed from the secondary sources (A and O) to the observation point. The resulting interference of the waves from the position of the observer, and hence the light intensity at this point, depends on this value.

Calculation of the first radius
We get that if the path difference is equal to half the light wavelength (λ / 2), then the light will come to the observer in antiphase. From this we can conclude that if the path difference is less than λ / 2, then the light will come in the same phase. This condition CA + AN-CH≤ λ / 2, by definition, is a condition that point A is in the first ring, that is, this is the first Fresnel zone. In this case, for the boundary of this circle, the path difference will be equal to half the light wavelength. So this equality allows us to determine the radius of the first zone, we denote it by P 1 . With a stroke difference corresponding to λ / 2, it will be equal to the segment OA. In the event that the CO distances significantly exceed the diameter of the hole (such options are usually considered), then, from geometric considerations, the radius of the first zone is determined by the following formula: P 1 = √ (λ * CO * OH) / (CO + OH).
Calculation of the radius of the Fresnel zone
The formulas for determining the subsequent values of the radii of the rings are identical to those considered above, only the number of the desired zone is added to the numerator. In this case, the equality of the difference in the course will have the form: CA + AN-CH≤ m * λ / 2 or CA + AN-SO-OH≤ m * λ / 2. It follows that the radius of the desired zone with the number "m" is determined by the following formula: P m = √ (m * λ * * ) / ( + ) = 1 √
Summary of Interim Results
It can be noted that the division into zones is the division of the secondary light source into sources having the same area, since P m = π * P m 2 - π * P m-1 2 = π * P 1 2 = P 1 . Light from neighboring Fresnel zones comes in the opposite phase, since the difference in the travel of a neighboring ring will, by definition, be equal to half the length of the light wave. Summarizing this result, we see that breaking holes into circles (such that light from neighboring ones comes to an observer with a fixed phase difference) will mean breaking into rings with the same area. This statement is easily proved using the problem.
Fresnel zones for a plane wave
Consider a breakdown of the area of the hole into thinner rings with equal area. These circles are secondary light sources. The amplitude of the light wave coming from each ring to the observer is approximately the same. In addition, the phase difference from the adjacent circle at point H is also the same. In this case, the complex amplitudes at the observer's point, when added together on a single complex plane, form part of a circle — an arc. The total amplitude is a chord. Now let us consider how the pattern of summing complex amplitudes changes in the case of a change in the radius of the hole, provided that the remaining parameters of the problem are preserved. In the event that the hole opens only one zone for the observer, the addition pattern will be represented by a part of the circle. The amplitude from the last ring will be rotated by an angle π relative to the central part, since the difference in the course of the first zone, according to the definition, is equal to λ / 2. This angle π will mean that the amplitudes are half the circle. In this case, the sum of these values at the observation point will be zero - zero length of the chord. If three rings are opened, then the picture will be one and a half circles and so on. The amplitude at the observer point for an even number of rings is zero. And in the case when an odd number of circles is used, it will be maximum and equal to the value of the diameter length on the complex plane of addition of amplitudes. The considered problems fully reveal the Fresnel zone method.

Briefly about special cases
Consider rare conditions. Sometimes when solving a problem it is said that a fractional number of Fresnel zones is used. In this case, a half ring is understood to mean a quarter of the circumference of the picture, which will correspond to half the area of the first zone. Similarly, any other fractional value is calculated. Sometimes a condition assumes that some fractional number of rings is closed, and so much is open. In this case, the total field amplitude is found as the vector difference of the amplitudes of the two problems. When all zones are open, that is, there are no obstacles to the passage of light waves, the picture will look like a spiral. It is obtained because when opening a large number of rings, one should take into account the dependence of the radiation emitted by the secondary source to the point of the observer and on the direction of the secondary source. We get that the light from the zone with a large number has a small amplitude. The center of the resulting spiral is in the middle of the circumference of the first and second rings. Therefore, the field amplitude in the case when all zones are open is half as much as when the first first circle is open, and the intensity differs by four times.
Fresnel zone light diffraction
Let's look at what they mean by this term. Fresnel diffraction is a condition when several zones open at once through an opening. If many rings are opened, then this parameter can be neglected, that is, we are approaching geometric optics. In the case when through the hole for the observer substantially less than one zone opens, this condition is called Fraunhofer diffraction. It is considered fulfilled if the light source and the observer's point are at a sufficient distance from the hole.
Comparison of lens and zone plate
If you close all the odd or even even Fresnel zones, then at the observer's point there will be a light wave with a larger amplitude. Each ring gives a half circle on a complex plane. So, if you leave the odd zones open, then only half of these circles will remain from the general spiral, which contribute to the total amplitude from bottom to top. An obstacle to the passage of a light wave in which only one type of ring is open is called a zone plate. The light intensity at the observer's point is many times higher than the light intensity on the plate. This is because the light wave from each open ring hits the observer in the same phase.
A similar situation is observed with the focusing of light using a lens. It, unlike the plate, does not close any rings, but shifts the light in phase by π * (+ 2 π * m) from those circles that are closed by the zone plate. As a result, the amplitude of the light wave doubles. Moreover, the lens eliminates the so-called mutual phase shifts that occur within one ring. It unfolds on a complex plane half the circle for each zone in a straight line segment. As a result, the amplitude increases by π times, and the entire spiral on the complex plane the lens unfolds in a straight line.