The definition of metacentric height can be formulated as follows. What is it? This is the distance between the center of gravity and the metacentre of the ship. The definition itself is not very clear, and therefore it is worth adding that very often this height is expressed through the stability of the vessel. This is because the metacentre is the main criterion for determining this very stability.
General concepts
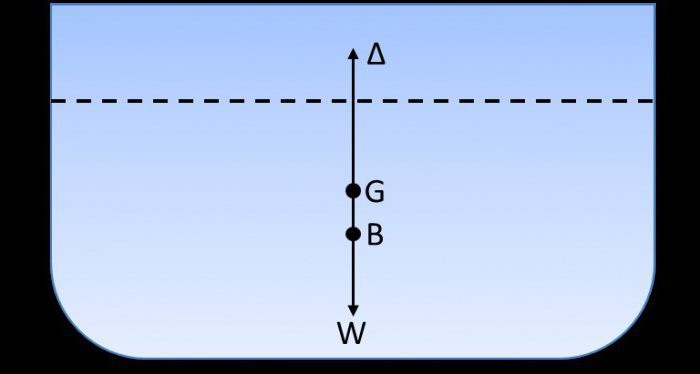
As previously mentioned, metacentric height is the elevation of the center of gravity above the metacentre of the ship itself. It is important to know that the greater the value of this characteristic, the greater will be the initial stability of the vessel. If for some reason this altitude deviates towards a negative value, this indicates that the vessel will not be able to sail without a bank. What does it mean? In no case can negative values of metacentric height be allowed. Although ... It is definitely impossible to get an exact answer to the question of whether a vessel having a negative value of this height will roll over. Since the theory of stability applies only to the inclinations of ships that do not exceed 10 degrees.
Rules and powers
It is important to note that there are rules of the Classification Communities that monitor the technical operation of ships. These documents describe that it is possible to operate only those ships whose metacentric height is at least 0.2 meters. To understand how a zero-height body will behave, one can imagine a keg floating in water. This parameter of this body will be 0, and its movement will occur along the longitudinal axis every time it is affected by any external force, for example, a wave or wind.
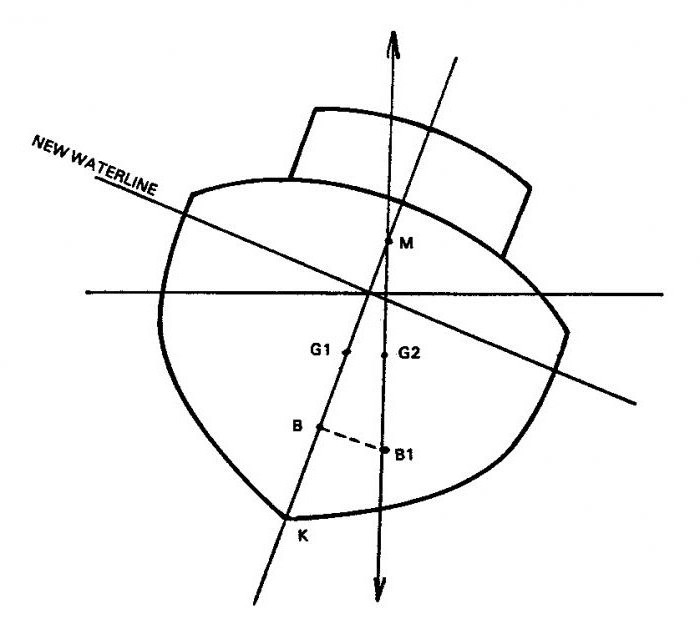
Another basis that allows a ship to sail is gravity. And also the Archimedean force. Naturally, gravity will pull the ship down, that is, to the bottom. The numerical value of this characteristic is equal to its weight, and it is applied to the center of gravity of the ship. The Archimedean force, or, as it is also called, the force of buoyancy, pushes a marine vessel out of the water. The strength of this effect is equal to the displacement of the ship, which is applied in the center of the underwater volume.
Work of forces
In the “direct” position of the vessel, it turns out that these two forces balance each other and are in the same vertical plane. Because of this, the ship is able to move through the water. In the event that a ship roll occurs, the center of the underwater volume of the CV is shifted toward the side of the ship. The displacement is due to the fact that the shape of the underwater part of the body changes. In addition, when the CV is shifted to one side, a restoring moment arises that will counteract the roll of the sea vessel. When a tilt occurs, the CV begins to turn around a point, which is conventionally called the metacentre m.
The distance from this conditional point of the metocenter m to the center of gravity of the vessel CT and will be its height. For example, for a regular rowboat, the numerical value of the metacentric height, which will be sufficient so that people can safely sit and stand, is 0.3 m. In principle, nothing complicated.
How to ensure stability
Knowing everything that was described above, the obvious question arises of how to evaluate the safety of a boat, sailing yacht, ship, etc.? How to understand how great the chances are for the ship to return from the “keel up” position to its normal, direct state?
In order to achieve this, it is necessary to improve the stability of the vessel. There are several proven methods for this. A sufficiently high stability can be ensured due to the fact that a fixed ballast will be placed on board the ship. However, it must be borne in mind that the center of gravity of the vessel will decrease with additional load. Shipbuilders, sailors, and anyone familiar with maritime affairs have this rule: every kilogram of cargo located under the waterline will increase the stability of the ship, but every kilogram above this line will worsen the position of the vessel.
Ship recovery
In order to increase the weight of, for example, a yacht, it is equipped with such a thing as a fixed ballast keel. But, it is important to note here that it can be placed only on the classic type of yacht. Any other species with such a keel will be too heavy. Classic yachts are the absolute stability of a river vessel, as it is called. The thing is that this category of ships can straighten up after almost any roll. The angle of heel, which is necessary so that the ship does not recover, is 155 degrees. This is a parameter of a yacht like Contessa 32. In other words, a river vessel of this class will be able to recover in a straight position even after it capsizes with a keel up.
It is important to understand that large vessels have great shape stability initially because of their size. Another important point is that the seawater should not get inside the ship when tilting through any hatches or holes. If this happens, the liquid on board can nullify all stability. This will happen due to the fact that the weight of the water hit makes the ship heavier. The metacentric height will be disturbed due to the displacement of the center of gravity. And the ship will begin to sink.
Ships with a superstructure
There is a type of ship that has a waterproof superstructure. Naturally, water will not be able to get inside such a ship, which means that stability will remain at the same level even with a large roll. This principle has become fundamental in the invention of tumbler lifeboats. There are life rafts and boats that are considered virtually non-tilting due to their construction. Such categories of ships are able to recover even after they are completely turned over.
You can take, for example, a sailing yacht, which has one tricky way to increase the stability of the vessel. The method is called peeling. And its essence lies in the fact that when tilting, the weight of the crew, ballast or swinging keel will move along the entire width of the vessel. Today, many different types of moving ballast are used. And also there is one newest one, which is the presence of underwater guided wings.
Experimental height
Further. In order to experimentally calculate the metacentric height of the ship, you can move a large load on the ship. The movement of cargo should occur at a certain distance Q from the place where it was originally located. Also, when moving an object, you need to measure a small angle of rotation, which is denoted as af. The numerical value of this characteristic will correspond to the angle of inclination of the ship.
This is how the transverse metacentric height in the formula will look:
h 0 = 0.525 (W / T) 2, m
B is the breadth of the vessel, which should be measured in meters, and T is the rolling period, determined in seconds.
It was this method of calculation, as well as the experimental method of determination, that became the main provisions that made it possible to take the height of the metacentre of the ship as the main criterion for its stability.
Sailing ships
Currently, sailing vessels are one of the most dangerous in terms of operation, as well as the most demanding for stability. The thing is that in the wind the sail of such a ship will be constantly exposed to strong air impact, which in such conditions will be the main point that creates the opportunity for roll. It is precisely because of the presence of a sail that ships with large and long masts and, as a result, large sails, need additional heavy stationary ballast, which will greatly reduce the center of gravity of the vessel, thereby creating a higher metacentric height.
It is very important to note: quite often they make such a mistake as assessing the stability of a ship only by its metacentre. Of course, this height will be the main criterion. However, one cannot ignore the benefits that are found throughout the static stability diagram. It includes not only the metacentre height.
Instability cases
There are three instances of ship instability. Let's consider them in more detail.
The first case arises in that situation if the height h> 0. This is due to the fact that the center of gravity is higher than the center of magnitude. If these conditions are met and the ship is tilted to either side, the line of action of the support force will cross the diametric plane higher than the center of gravity.
The second case of instability will occur when the metacentric height is zero. In this case, practically, as in the previous one, the center of gravity will be higher than the center of magnitude. And when the ship is tilted, it will happen that the center line will run along the line of magnitude. In this case, the center of magnitude will always be located in the same vertical line with the center of gravity. With this arrangement of forces, the reconstructing pair that aligns the ship will simply be absent. Without the influence of any external forces, the ship will not be able to return to its original, direct position.
The third case arises if h <0. In this case, the center of gravity will be higher than the center of magnitude, and in an inclined position the line of action of the support force will stop the trace of the diametrical plane below the center of gravity. In this case, at the slightest tilt, a negative pair of forces will form, affecting the vessel and leading to its capsizing.