For many people, mathematical analysis is just a collection of obscure numbers, icons, and definitions that are far from real life. However, the world in which we exist is built on numerical laws, the identification of which helps not only to know the world around and solve its complex problems, but also to simplify everyday practical tasks. What does a mathematician mean when he says that a numerical sequence converges? This should be discussed in more detail.
What is infinitesimal ?
Imagine matryoshkas that fit one into another. Their sizes, recorded in the form of numbers, starting with the larger and ending with the smaller of them, form a sequence. If you imagine an infinite number of such bright figures, the resulting series will be fantastically long. This is a convergent numerical sequence. And she tends to zero, since the size of each subsequent nesting dolls, catastrophically decreasing, gradually turn into nothing. Thus, one can easily explain: what is infinitesimal.
A similar example would be a road that goes into the distance. And the visual dimensions of the car leaving it from the observer, gradually shrinking, turn into a shapeless speck that resembles a dot. Thus, the machine, as an object, moving away in an unknown direction, becomes infinitely small. The parameters of the indicated body will never be zero in the literal sense of the word, but invariably tend to this value in the final limit. Therefore, this sequence converges again to zero.
Calculate everything drop by drop
Imagine the everyday situation. The doctor prescribed the patient to take the medicine, starting with ten drops per day and adding two in each subsequent day. And so the doctor suggested continuing until the contents of the vial with the medicine, the volume of which is 190 drops, are over. It follows from the foregoing that the number of those scheduled by day will be the following number series: 10, 12, 14, and so on.
How to find out the duration of the entire course and the number of members in the sequence? Here, of course, you can count the drops in a primitive way. But it’s much easier, given the regularity, to use the arithmetic progression sum formula with a step of d = 2. And using this method, find out that the number of members of the number series is 10. Moreover, a is 10 = 28. The member number indicates the number of days the medicine was taken, and 28 corresponds to the number of drops that the patient should take on the last day. Does this sequence converge? No, because, despite the fact that from below it is limited to 10, and from above to 28, such a number series has no limit, unlike previous examples.
What is the difference?
Now let’s try to clarify: when a number series turns out to be a convergent sequence. A definition of this kind, as can be concluded from the above, is directly related to the concept of the final limit, the presence of which reveals the essence of the issue. So what is the fundamental difference between the earlier examples? And why in the last of them the number 28 cannot be considered the limit of the number series X n = 10 + 2 (n-1)?
To clarify this question, we consider another sequence given by the formula below, where n belongs to the set of natural numbers.
This community of members is a set of ordinary fractions, the numerator of which is 1, and the denominator is constantly increasing: 1, ½ ...
Moreover, each subsequent representative of this series in their location on the number line is increasingly approaching 0. And this means that there is such a neighborhood where the points are clustered around zero, which is the limit. And the closer they are to it, the denser their concentration on the number line becomes. And the distance between them is catastrophically reduced, turning into infinitesimal. This is a sign that the sequence is converging.
Similarly, the multi-colored rectangles depicted in the figure, when removed in space, are visually more crowded, turning into negligible in the hypothetical limit.
Infinitely large sequences
Having examined the definition of a convergent sequence, we now turn to the opposite examples. Many of them have been known to man since ancient times. The simplest versions of divergent sequences are rows of natural and even numbers. They are differently called infinitely large, since their members, constantly increasing, are increasingly approaching positive infinity.
Examples of such can also be any of the arithmetic and geometric progressions with a step and denominator, respectively, greater than zero. In addition, numerical series are considered divergent sequences, which have no limit at all. For example, X n = (-2) n -1 .
Fibonacci sequence
The practical use of the numerical series indicated above for mankind is undeniable. But there are so many other great examples. One of them is the Fibonacci sequence. Each of its members, which begin with a unit, represents the sum of the previous ones. Its first two representatives are 1 and 1. The third 1 + 1 = 2, the fourth 1 + 2 = 3, the fifth 2 + 3 = 5. Further, according to the same logic, numbers 8, 13, 21 and so on follow.
This series of numbers increases unlimitedly and does not have a finite limit. But he has one more remarkable property. The ratio of each previous number to the next is increasingly approaching in value to 0.618. Here you can understand the difference between a converging and diverging sequence, because if you make a series of the quotient fractions obtained, the specified numerical order will have a finite limit of 0.618.
Fibonacci coefficient sequence
The above number series is widely used for practical purposes for technical analysis of markets. But this is not limited to his capabilities, which the Egyptians and Greeks knew and knew how to put into practice in ancient times. This is proved by the pyramids they built and the Parthenon. After all, the number 0, 618 is a constant coefficient of the well-known in ancient times golden section. According to this rule, any arbitrary segment can be divided so that the ratio between its parts will coincide with the ratio between the largest of the segments and the total length.
We construct a series of these relations and try to analyze this sequence. The number series will be as follows: 1; 0.5; 0.67; 0.6; 0.625; 0.615; 0.619 and so on. Continuing in this way, one can verify that the limit of the convergent sequence will indeed be 0.618. However, it is necessary to note other properties of this pattern. Here the numbers seem to be different, and not at all in ascending or descending order. This means that this convergent sequence is not monotonic. About why this will be discussed further.
Monotony and limitation
Members of a number series with increasing numbers can clearly decrease (if x 1 > x 2 > x 3 > ...> x n > ...) or increase (if x 1 <x 2 <x 3 <... <x n <...). In this case, the sequence is called strictly monotonic. Other patterns may also be observed where the number series will be non-decreasing and non-increasing (x 1 ≥x 2 ≥x 3 ≥ ... ≥x n ≥ ... or x 1 ≤x 2 ≤x 3 ≤ ... ≤x n ≤ ...), then converging sequentially monotonous, too, only not in the strict sense. A good example of the first of these options is the number series given by the following formula.
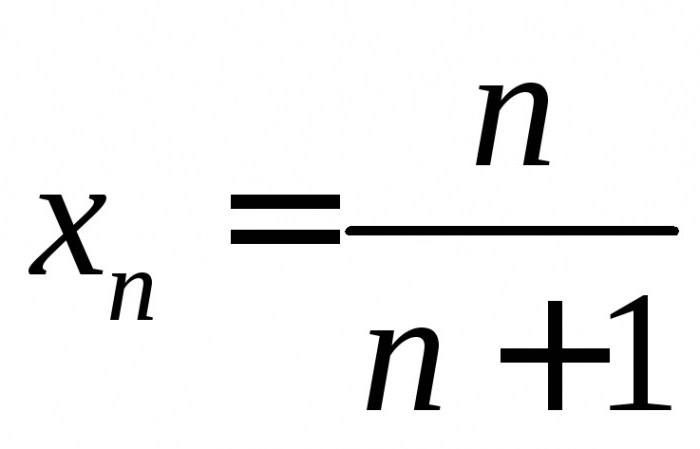
Having written the numbers of this series, you can see that any of its members, unlimitedly approaching 1, will never exceed this value. In this case, one speaks of the boundedness of the convergent sequence. This happens whenever a positive number M is found that is always larger than any of the members of the series modulo. If the number series has the signs of monotony and has a limit, and therefore converges, then it is necessarily endowed with this property. Moreover, the converse does not have to be true. This is evidenced by the theorem on the boundedness of a convergent sequence.
The application of such observations in practice is very useful. We give a concrete example by examining the properties of the sequence X n = n / n + 1 and prove its convergence. The fact that it is monotonous is easy to show, since (x n +1 - x n ) is a positive number for any values of n. The limit of the sequence is equal to the number 1, which means that all the conditions of the above theorem, also called the Weierstrass theorem, are satisfied. The boundedness theorem of a convergent sequence states that if it has a limit, then in any case it turns out to be bounded. However, we give the following example. The number series X n = (-1) n is bounded below by a number of -1 and from above 1. But this sequence is not monotonic, has no limit and therefore does not converge. That is, limitation does not always imply a limit and convergence. For this to be carried out, the coincidence of the lower and upper limits, as in the case of Fibonacci ratios, is necessary.
Numbers and laws of the universe
The simplest versions of a convergent and divergent sequence are, perhaps, the numerical series X n = n and X n = 1 / n. The first of them is a natural series of numbers. She is, as already mentioned, infinitely large. The second convergent sequence is bounded, and its terms in magnitude approach infinitesimal. Each of these formulas represents one of the sides of the multifaceted Universe, helping a person in the language of numbers and signs to imagine and calculate something unknowable, inaccessible to limited perception.
The laws of the universe, starting from negligible and ending with incredibly large, are also expressed by the golden coefficient 0.618. Scientists believe that it is the foundation of the essence of things and is used by nature to form its parts. The relations already mentioned by us between the subsequent and previous members of the Fibonacci series do not complete the demonstration of the amazing properties of this unique series. If we consider the quotient of dividing the previous term into the next one through one, then we get the row 0.5; 0, 33; 0.4; 0.375; 0.384; 0.380; 0.382 and so on. It is interesting that this limited sequence converges, it is not monotonous, but the ratio of the neighboring numbers extreme from a certain term always turns out to be approximately 0.382, which can also be used in architecture, technical analysis, and other industries.

There are other interesting coefficients of the Fibonacci series, they all play a special role in nature, and are also used by humans for practical purposes. Mathematicians are sure that the Universe is developing in a kind of “golden spiral” formed from the indicated coefficients. With their help, it is possible to calculate many phenomena occurring on Earth and in space, starting from the growth of the number of certain bacteria and ending with the movement of distant comets. Similar laws obey, as it turns out, the DNA code.
Decreasing geometric progression
There is a theorem that states the uniqueness of the limit of a convergent sequence. This means that it cannot exist two or more limits, which is undoubtedly important for finding its mathematical characteristics.
Let's consider some cases. Any number series composed of members of an arithmetic progression is divergent, except in the case with a zero step. The same applies to geometric progression, the denominator of which is greater than 1. The limits of such numerical series are the “plus” or “minus” of infinity. If the denominator is less than -1, then there is no limit at all. Other options are possible.
Consider the number series given by the formula X n = (1/4) n -1 . At first glance it is easy to understand that this converging sequence is limited, because it is strictly decreasing and in no way is able to take negative values.
We will write a certain number of its members in a row.
It will turn out: 1; 0.25; 0.0625; 0.015625; 0.00390625 and so on. Simple calculations are enough to understand how quickly a given geometric progression from the denominators 0 <q <1 decreases. While the denominator of the members grows indefinitely, they themselves become infinitesimal. This means that the limit of the number series is 0. This example once again demonstrates the limitations of a convergent sequence.
Fundamental sequences
Augustine Louis Cauchy, a French scientist, showed the world many works related to mathematical analysis. He gave definitions to such concepts as differential, integral, limit and continuity. He also investigated the basic properties of convergent sequences. In order to understand the essence of his ideas, it is necessary to summarize some important details.
At the very beginning of the article it was shown that there are sequences for which there is a neighborhood where the points depicting the members of a certain series on the number line begin to condense, lining up more and more tightly. At the same time, the distance between them decreases as the number of the next representative increases, turning into infinitesimal. Thus, it turns out that in this neighborhood an infinite number of representatives of this series are grouped, while outside of them there are a finite number. Such sequences are called fundamental.
The famous Cauchy criterion, created by a French mathematician, clearly indicates that the presence of such a property is sufficient to prove that the sequence converges. The converse is also true.
It should be noted that this conclusion of the French mathematician is for the most part purely theoretical interest. Its practical application is considered rather complicated, therefore, to determine the convergence of series, it is much more important to prove the existence of a finite limit for a sequence. Otherwise, it is considered diverging.
When solving problems, one should also take into account the basic properties of convergent sequences. They are presented below.
Infinite amounts
Such famous ancient scholars as Archimedes, Euclid, Eudox used the sums of infinite numerical series to calculate the lengths of curves, volumes of bodies and areas of figures. In particular, it was in this way that we managed to find out the area of the parabolic segment. For this, the sum of the numerical series of the geometric progression with q = 1/4 was used. In a similar way, the volumes and areas of other arbitrary figures were found. This option was called the "exhaustion" method. The idea was that the investigated body with a complex shape was divided into parts, which were figures with easily measured parameters. For this reason, it was not difficult to calculate their area and volume, then they folded.

By the way, similar tasks are very familiar to modern students and are found in the tasks of the exam. A unique method found by distant ancestors is by far the easiest solution. Even if the parts into which the numerical figure is divided are only two or three, the addition of their areas is still the sum of the number series.
Much later, the ancient Greek scholars Leibniz and Newton, based on the experience of wise predecessors, learned the laws of integral calculation. Knowledge of the properties of sequences helped them solve differential and algebraic equations. Currently, the theory of series created by the efforts of many generations of talented scientists gives a chance to solve a huge number of mathematical and practical problems. And the study of numerical sequences is the main task solved by mathematical analysis from the moment of its creation.