Inequalities and systems of inequalities is one of the topics that is taught in algebra in high school. In terms of complexity, it is not the most difficult, because it has simple rules (about them a little later). As a rule, students learn to solve inequality systems quite easily. This is also due to the fact that teachers simply "train" their students on this topic. And they cannot help but do this, because it is studied further with the use of other mathematical quantities, and is also checked for OGE and EGE. In school textbooks, the topic of inequalities and systems of inequalities is disclosed in great detail, so if you are going to study it, it is best to resort to them. This article only retells large materials, and there may be some omissions.
The concept of a system of inequalities
If we turn to the scientific language, we can define the concept of "system of inequalities." This is a mathematical model that presents several inequalities. Of course, a solution is required from this model, and its quality will be the general answer for all the inequalities of the system proposed in the task (usually they write in it, for example: "Solve the system of inequalities 4 x + 1> 2 and 30 - x > 6 ... "). However, before moving on to the types and methods of solutions, you still need to understand something else.
Systems of inequalities and systems of equations
In the process of studying a new topic, misunderstandings very often arise. On the one hand, everything is clear and most likely I want to start solving tasks, and on the other, some moments remain in the “shadow” and are not well understood. Also, some elements of already acquired knowledge may be intertwined with new ones. As a result of this “overlay” errors often occur.

Therefore, before proceeding with the analysis of our topic, we should remember about the differences between equations and inequalities, their systems. To do this, we need to clarify again what these mathematical concepts are. An equation is always equality, and it is always equal to something (in mathematics this word is denoted by the sign "="). Inequality, on the other hand, is a model in which one quantity is either greater or less than the other, or contains the assertion that they are not the same. Thus, in the first case it is appropriate to talk about equality, and in the second, no matter how obvious it sounds from the name itself, about the inequality of the source data. The systems of equations and inequalities practically do not differ from each other and the methods for solving them are the same. The only difference is that in the first case, equalities are used, and in the second, inequalities are used.
Types of Inequalities
Two types of inequalities are distinguished: numerical and with an unknown variable. The first type is the provided values (numbers) that are unequal to each other, for example, 8> 10. The second is inequalities containing an unknown variable (indicated by some letter of the Latin alphabet, most often X). This variable needs to be found. Depending on how many of them, inequalities are distinguished in the mathematical model from one (make up a system of inequalities with one variable) or several variables (make up a system of inequalities with several variables).
The last two types according to their degree of construction and level of complexity of the decision are divided into simple and complex. Simple are also called linear inequalities. They, in turn, are divided into strict and non-strict. The strict ones specifically “say” that one quantity must necessarily be either less or more, therefore this is pure inequality. Several examples can be given: 8 x + 9> 2, 100 - 3 x> 5, etc. Non-strict ones also include equality. That is, one quantity may be greater than or equal to another quantity (sign "≥") or less than or equal to another quantity (sign "≤"). Even in linear inequalities, the variable is not in the root, the square, is not divided into anything, because of what they are called "simple". Complex ones include unknown variables, the finding of which requires more mathematical operations. They are often located in a square, cube or under the root, they can be modular, logarithmic, fractional, etc. But as our task becomes to understand the solution of systems of inequalities, we will talk about a system of linear inequalities. However, before that, a few words should be said about their properties.
Inequality Properties
The following points belong to the properties of inequalities:
- The inequality sign is reversed if the operation of changing the sequence of the parties is used (for example, if t 1 ≤ t 2 , then t 2 ≥ t 1 ).
- Both parts of the inequality allow us to add the same number to ourselves (for example, if t 1 ≤ t 2 , then t 1 + number ≤ t 2 + number).
- Two or more inequalities that have the sign of the same direction can add their left and right sides (for example, if t 1 ≥ t 2 , t 3 ≥ t 4 , then t 1 + t 3 ≥ t 2 + t 4 ).
- Both parts of the inequality allow themselves to be multiplied or divided by the same positive number (for example, if t 1 ≤ t 2 and number ≤ 0, then the number · t 1 ≥ the number · t 2 ).
- Two or more inequalities having positive terms and a sign of the same direction allow you to multiply yourself by each other (for example, if t 1 ≤ t 2 , t 3 ≤ t 4 , t 1 , t 2 , t 3 , t 4 ≥ 0 then t 1 · t 3 ≤ t 2 · t 4 ).
- Both parts of the inequality allow themselves to be multiplied or divided by the same negative number, but the sign of the inequality changes (for example, if t 1 ≤ t 2 and number ≤ 0, then the number · t 1 ≥ the number · t 2 ).
- All inequalities have the property of transitivity (for example, if t 1 ≤ t 2 and t 2 ≤ t 3 , then t 1 ≤ t 3 ).
Now, after studying the basic principles of the theory related to inequalities, we can proceed directly to the consideration of the rules for solving their systems.
Solution of systems of inequalities. General information. Solutions
As mentioned above, the solution is the values of the variable that are suitable for all the inequalities of the given system. The solution of systems of inequalities is the implementation of mathematical actions, which ultimately lead to the solution of the entire system or prove that it has no solutions. In this case, they say that the variable refers to an empty numerical set (written like this: the letter denoting the variable ∈ (the sign "belongs") ø (the sign "empty set"), for example, x ∈ ø (read like this: "Variable" x " belongs to the empty set "). There are several ways to solve systems of inequalities: graphical, algebraic, and the method of substitution. It is worth noting that they relate to those mathematical models that have several unknown variables. In the case where there is only one, the interval method is suitable.
Graphical way
Allows you to solve a system of inequalities with several unknown quantities (from two or more). Thanks to this method, the system of linear inequalities is solved quite easily and quickly, so it is the most common way. This is because the construction of the graph reduces the amount of writing mathematical operations. It becomes especially pleasant to get a little distracted from the pen, pick up a pencil with a ruler and start further actions with their help when a lot of work is done and you want a little variety. However, some people do not like this method because they have to break away from the task and switch their mental activity to drawing. However, this is a very effective way.
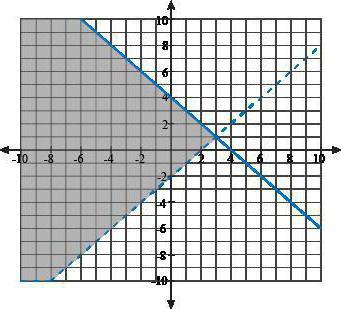
In order to solve the system of inequalities using the graphical method, it is necessary to transfer all members of each inequality to their left side. The signs will be reversed, zero should be written on the right, then each inequality must be written separately. As a result, the inequalities yield functions. After that, you can get a pencil and a ruler: now you need to draw a graph of each received function. The whole set of numbers that appears in the interval of their intersection will be a solution to the system of inequalities.
Algebraic way
Allows you to solve a system of inequalities with two unknown variables. Inequalities must also have the same sign of inequality (that is, they must contain either only the sign “more” or only the sign “less”, etc.) Despite its limitations, this method is also more complicated. It is applied in two stages.
The first includes actions to get rid of one of the unknown variables. First you need to select it, then check for the presence of numbers in front of this variable. If they are not (then the variable will look like a single letter), then nothing is changed, if there is (the type of the variable will be, for example, 5y or 12y), then it is necessary to make sure that in each inequality the number before the selected variable is the same. To do this, multiply each term of the inequality by a common factor, for example, if 3y is written in the first inequality and 5y is written in the second, then all the terms of the first inequality must be multiplied by 5, and the second by 3. We get 15y and 15y, respectively.
The second stage of the decision. It is necessary to transfer the left side of each inequality to their right side with the opposite sign of each term, write zero on the right. Then the most interesting thing comes: getting rid of the selected variable (otherwise called "reduction") during the folding of inequalities. This results in a single variable inequality that needs to be addressed. After that, the same thing should be done, only with another unknown variable. The results obtained will be the solution to the system.
Substitution Method
Allows you to solve the system of inequalities if possible, introduce a new variable. Usually this method is used when an unknown variable in one member of the inequality is raised to the fourth power, and in the other member it has a square. Thus, this method is aimed at reducing the degree of inequality in the system. The inequality of the sample x 4 - x 2 - 1 ≤ 0 in this way is solved as follows. A new variable is introduced, for example, t. They write: "Let t = x 2 ", then the model is rewritten in a new form. In our case, we get t 2 - t - 1 ≤0. This inequality must be solved by the interval method (about it a little later), then back to the variable X, then do the same with the other inequality. The responses received will be the solution to the system.
Interval Method
This is the easiest way to solve inequality systems, and at the same time, it is universal and widespread. It is used in high school, and even in higher education. Its essence lies in the fact that the student is looking for intervals of inequality on the number line, which is drawn in a notebook (this is not a graph, but just an ordinary line with numbers). Where the gaps of inequalities intersect, a solution to the system is found. To use the interval method, you must complete the following steps:
- All members of each inequality are transferred to the left side with the opposite sign (zero is written on the right).
- Inequalities are written out separately, the decision of each of them is determined.
- The intersections of inequalities on the number line are found. All numbers at these intersections will be a solution.
Which way to use?
Obviously the one that seems the easiest and most convenient, but there are times when tasks require a certain method. Most often, they say that they need to be solved either using a schedule or by the interval method. The algebraic method and substitution are rarely used or are not used at all, since they are quite complex and confusing, and moreover they are more applicable for solving systems of equations, rather than inequalities, so you should resort to drawing graphs and intervals. They bring clarity, which cannot but contribute to the efficient and quick implementation of mathematical operations.
If something fails
During the study of a particular topic in algebra, naturally, problems may arise with its understanding. And this is normal, because our brain is designed so that it is not able to understand complex material at a time. Often you need to re-read a paragraph, take the help of a teacher, or take up practice in solving typical tasks. In our case, they look, for example, like this: "Solve the system of inequalities 3 x + 1 ≥ 0 and 2 x - 1> 3." Thus, personal aspiration, the help of outsiders and practice help in understanding any complex topic.
Researcher?
And it’s also a very good solution, but not for writing off homework, but for self-help. In them, you can find systems of inequalities with a solution, look at them (as patterns), try to understand how the author of the solution coped with the task, and then try to do this on his own.
conclusions
Algebra is one of the most difficult subjects in the school. Well, what can you do? Mathematics has always been like this: for some it is easy, but for someone with difficulty. But in any case, it should be remembered that the general educational program is designed so that any student can handle it. In addition, one must keep in mind a huge number of assistants. Some of them were mentioned above.