One of the laws of hydrostatics is the Archimedes rule. In the article we will tell you what it is, we will derive its formula. Consider what forces act on the body when it is completely and partially immersed in a liquid. We will tell a story that helped Archimedes make his famous discovery.
Body immersion in liquid
Before moving on to the law of hydrostatics, we will conduct an experiment. We will weigh the body, for example, a bar or a piece of plasticine, using a dynamometer.
Weight is the force the body exerts on a suspension or support. In our case, the suspension is the hook of the dynamometer. The division price of the device is 0.05 Newton (N). We will hang the body to it and see on the scale how much it weighs. The device displays a value of 1 N.
If the dynamometer shows a force of one Newton, then it is pulled up by a force equal to one Newton (spring elastic force). Denote it with the letter F. The body is in equilibrium, but what balances F? The force of gravity. It is attached to the center of gravity and is directed downward. F heavy = F = 1 N.
Take a glass of water and gradually immerse the body in it (see the figure above). What happens to a dynamometer? As soon as the body just touched the surface of the water, the dynamometer already shows a lower value (before diving - figure a, after - figure b). The deeper the body sinks, the less the dynamometer readings become. When the whole body is in water, on the scale of the device we will see a value of 0.2 Newton.
Archimedean force
To understand the law of hydrostatics, a scheme will help us. We will depict a dynamometer and a body in fluid.
The spring of the device is stretched, as we found out, by a force of 0.2 N. Denote it by F ′. It is still directed upwards because the dynamometer spring is taut. When we plunged the body into a fluid, did the gravity acting on it change? No, the earth still attracts this body. Let us show on the diagram this force with the same vector as before.
And why, then, the readings of the dynamometer decreased? In addition to the gravity and elasticity of the spring, now the upward pushing force Fout exerts on the body from the water side. It is also called Archimedean (F A ).
Find out what in our case it is equal. To do this, we write down the equilibrium condition: upward F ′ and Foutrev together are balanced by gravity F heavy . F ′ + F A = F F A = F heavy - F ′. We establish by this formula what the strength of Archimedes is equal to. F A = 1 - 0.2 = 0.8 N. We conducted the experiment, and now we will explain why this happens, what is the nature of this force.
Deep pressure
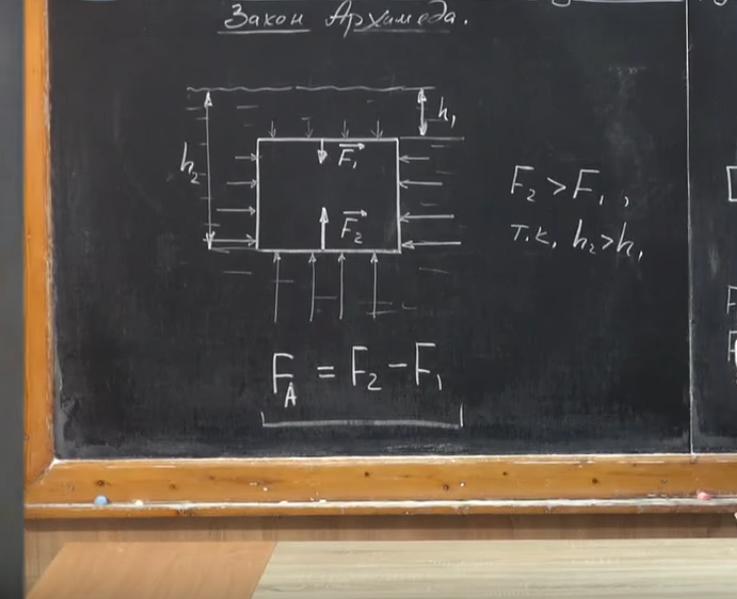
Imagine a liquid in which the body is completely immersed. In the depths it is compressed, there is pressure, which is called hydrostatic. Its value depends on the depth and density of the liquid. A body in space takes up some volume. Its upper part is at a shallower depth, which means that the hydrostatic pressure there will be less than below. The greatest pressure is exerted on the lower body.
To find the force of influence, it is necessary to multiply the pressure by the surface area of the body. If the pressure above is less, then the force will be small. Denote it by F 1 . The force acting on the bottom surface is F 2 . F 2 > F 1 , because h 2 (depth at the bottom of the bar)> h 1 (depth of the upper body).
Pressure forces also act on the sides of the item. But since they are the same and directed in different directions, they cancel each other out. The resultant F 1 and F 2 can be found by subtracting a smaller force from a larger one. F = F 2 - F 1 . F is directed upward, because the resultant of the opposing forces always has the same vector as the larger one. It will be impossible to deduce the formula of the law of hydrostatics without this understanding.
The resultant F is the Archimedean force. F A = F 2 - F 1 . Why is there buoyancy force? With increasing depth, the fluid pressure increases. If you take the atmospheric, it also depends on the height. Every 12 m, it decreases by 1 mmHg. That is why the balloon always tends to go up.
Ejection force calculation
Not only the Archimedes rule is one of the basic laws of hydrostatics. Pascal's law also applies to them. We will use it to derive a formula for finding the Archimedean force if the body is not completely immersed in the liquid, but partially. Suppose we have the same body in the shape of a rectangular parallelepiped, and it is immersed in a liquid. The area of the base of the body is denoted by the letter S, and the depth to which the body is immersed, by the letter h. We will draw a diagram that will help us make the calculation.
What forces act on the body? Above is atmospheric pressure. The impact is denoted by P 1 . P 1 = P atm . The pressure at depth will be called P 2 . What is it equal to? Atmospheric pressure also acts on the surface of the liquid. If it did not exist, then P 2 would be just a hydrostatic effect, which is calculated by the formula P = ρ * g * h. But there is still atmospheric pressure. Pascal's law in hydrostatics states that the effect on the liquid is transmitted to all its points, and this happens without change. Atmospheric pressure is added to hydrostatic. Therefore, P 2 = P atm + ρ * g * h.
Now we can find the force of pressure. F 1 presses on top of the body, F 2 on the bottom. The resultant of these forces will be Archimedean. F 1 = P 1 * S or F 1 = P atm * S. F 2 = P 2 * S or F 2 = (P atm + ρ * g * h) * S. F A = F 2 - F 1 . Substitute data. F A = P atm * S + ρ * g * h * S - P atm * S. Reduce P atm * S. This means that it does not matter what the atmospheric pressure is, the buoyancy force does not depend on it. But which indicator is important? This expression h * S is the volume of the submerged part of the body. Denote it by V bur .
Archimedes Experience
The basic law of hydrostatics is Archimedes' rule: if you immerse the body in a liquid, it will displace a volume equal to the part of the body that is below the surface.
In Greece, King Hieron ruled. He ordered a gold crown to the jeweler to donate it to the temple. He gave the master a bar of gold, from which he made a crown. After a while, rumors reached Hieron that the jeweler had deceived him, replaced part of the metal with silver. The king invited Archimedes and asked him to check whether this was so.
Archimedes went to the bathhouse to freshen up. I must say that in ancient Greece the bath was not a steam room, but a bathtub filled to the brim with cool water. Entering it, the scientist noticed that part of the liquid spilled out. Moreover, the deeper Archimedes sank, the more water was on the floor. So the discovery was made that the amount of displaced fluid is equal to the volume of the submerged body. V burr = V ext .

Archimedes conducted the following experiment. He took a bar of gold of the same mass as the crown, and a bar of silver of similar weight. Archimedes immersed these ingots in a liquid. It turned out that silver displaces more water than gold. And when he plunged a crown of the same mass, it turned out that it displaces more liquid than a gold ingot, but less than a silver one. From this Archimedes concluded that the jeweler was unclean and added silver to the crown. He told Hieron about this, and he gave Archimedes a crown as a reward. What happened to the jeweler, the story is silent.
Archimedes Law
Back to the formula. Having made some transformations, we obtain F A = ρ * g * V burial . What is ρ * V burial ? This is the mass of the displaced fluid. If we multiply it by the acceleration of gravity (ρ * g * V burr ), then we recognize the force of gravity acting on the displaced fluid. But since the latter is motionless, it will be its weight.
Now we know that power is a vector that has a direction. She is directed up. The vector module is equal to the weight of the fluid displaced by the body. Based on this, one can formulate the Archimedes hydrostatic law: a force that is lowered into the liquid acts on a body equal to the weight of the liquid displaced by this body. This rule is also called the principle of crowding out.